Determining the earth's size
By the
fifth century BCE, the Greeks had firmly established that the earth was a sphere.
Although they knew it was a sphere, they didn't know how big the sphere was.
The
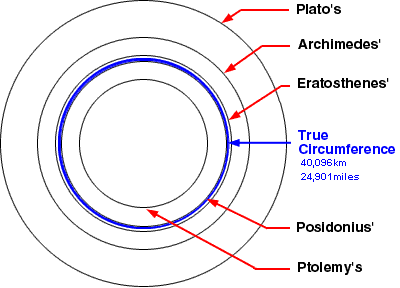
philosopher Plato (400 BCE) declared the earth's circumference to be 64,412 kilometers
(40,000 miles). Some 150 years later, the mathematician Archimedes estimated it
to be 48,309 kilometers (30,000 miles). It's not known exactly how Plato or
Archimedes arrived at their calculations, but Plato's measurement was off by
sixty percent and Archimedes' by twenty percent. At least they were making
progress.
Observations
and calculations by two later Greeks, Eratosthenes and Posidonius,
finally resulted in accurate estimates of the size of the earth.
In the
third century BCE, Eratosthenes, a Greek librarian in Alexandria, Egypt, determined the earth's
circumference to be 40,250 to 45,900 kilometers (25,000 to 28,500 miles) by
comparing the Sun's relative position at two different locations on the earth's
surface. Because of differences in translations or interpretations of his
records, and his own methodological errors, the exact figures are in dispute.
Today, the earth's circumference is usually accepted to be 40,096 kilometers
(24,901 miles). If you take the lowest estimate attributed to Eratosthenes, his
error was less than one percent—a phenomenal calculation.
Eratosthenes' methods
Eratosthenes
determined the earth's size by observing known phenomena and applying basic
arithmetic and geometry to them. Here's how he did it.
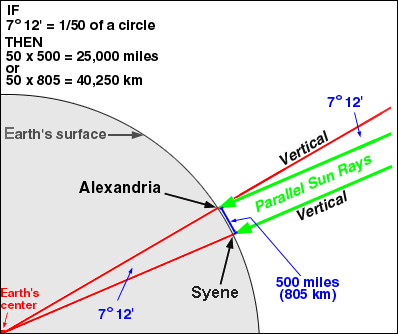
Eratosthenes' methods
were simple but effective. All he needed to know was the distance between two
locations and what percentage of a circle this distance constituted.
While in Syene, Egypt (known today as Aswan), he noticed that the sun's rays
shone directly down a well, casting no shadow at all. From this, he concluded
that the sun was directly overhead at Syene. On the
same date in Alexandria, a rod perpendicular to the ground
cast a shadow that was 7° 12' from perpendicular.
Eratosthenes
then divided 360° by 7° 12' and determined that 7° 12' was 1/50th of a circle.
Now all he had to do was find the distance from Syene
to Alexandria and multiply it by 50 to get the
earth's circumference.
Many
scholars believe Eratosthenes measured the distance by measuring a single pace
and then counting the number of paces from Syene to Alexandria. While this is possible, it is just
as likely that he counted the revolutions of a wheel with a known
circumference, since this was a common method of measurement in both Egypt and Greece. Either way, he probably hired
someone or enlisted a slave to accomplish the task. The distance figure he used
was 805 kilometers or 500 miles.
Next, he
multiplied this distance by 50 to get 40,250 kilometers (25,000 miles). Today,
most scientists set the earth's circumference at 40,096 kilometers (24,901
miles). This gives Eratosthenes' estimate less than a one percent error—an
excellent approximation of the earth's circumference.
Eratosthenes'
errors
Syene is not on the Tropic of Cancer, where the sun's
rays are directly overhead during the summer solstice. It is actually 37
kilometers (23 miles) north of the Tropic of Cancer.
Alexandria is not due north of Syene and the distance between them is not 805 kilometers.
The actual distance corresponds to an angular measurement not of 7° 12', but
rather of 7° 30'.
All in all,
it's amazing that his calculations came as close as they did to the earth's
true circumference.
Posidonius
used the stars to determine the earth's circumference. He observed that a given
star could be seen just on the horizon at Rhodes. He then measured the star's elevation
at Alexandria, Egypt, and calculated the angle of
difference to be 7.5 degrees or 1/48th of a circle. Multiplying 48 by what he
believed to be the correct distance from Rhodes to Alexandria (805 kilometers
or 500 miles), Posidonius calculated the earth's
circumference to be 38,647 kilometers (24,000 miles)—an error of only three
percent. The exact details of his methods are not known, but we do know the
distance he used was incorrect and the fact that Rhodes is not due north of Alexandria would result in computational
errors. Although his measurements were flawed, he was lucky because the errors
canceled themselves out and he arrived at a fairly accurate calculation of the
earth's circumference.
In the
second century CE (Common Era) in Alexandria, a philosopher named Claudius Ptolemaeus, or Ptolemy, revised Posidonius'
calculations and set the earth's circumference at 28,985 kilometers (18,000
miles)—an error of nearly 28 percent. Because of Ptolemy's contributions to
geography and cartography, his circumference was used throughout the
Renaissance. It is believed that Christopher Columbus used Ptolemy's number to
plan his voyage to the Far East. Everyone knows how that voyage ended—Columbus bumped into a continent that was
hiding in the 28 percent error.
With the
circumnavigation of the globe and subsequent scientific calculations, the
accepted value of the circumference of the earth returned from Ptolemy's
calculation back to that of Eratosthenes. After countless millennia,
scientists, explorers, clergy, and laymen finally knew the "true
shape" and "true size" of the earth. Of course, this geographic
euphoria wouldn't last.
Refining the earth's shape
It seems
that nothing is perfect, and that goes for the earth's shape as well. As
explorers and scientists of the past investigated the earth more closely, they
realized that it is not a perfect sphere but an ellipsoid—and an imperfect one
at that.

