The effect of the light source on the projection plane
In previous
examples, the location for our theoretical light source was assumed to be the
center of the earth. But the light source for a map projection can be
positioned at three different locations, resulting in three different
perspectives for the map projection.
·
Center
of the earth
·
Opposite
side of the earth from the map projection
·
Infinite
location in space
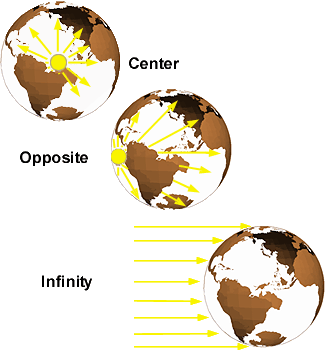
Most map projections use the center of the earth as their light source. Some projections are pure mathematical constructs and the concept of a light source doesn't really apply.
For conic
and cylindrical map projections, the light source is usually the center of the
earth. It is planar projections that are most affected by light source origin.
Different light sources are commonly used to achieve different effects and
these effects are most evident on the polar projections.
The graphic below illustrates three different planar map projections and how three different perspectives affect meridians and parallels. All three are still azimuthal (planar) but, as you can see, the distance between the parallels varies greatly between them. In the case of the Gnomonic projection, many of the parallels are not displayed at all.
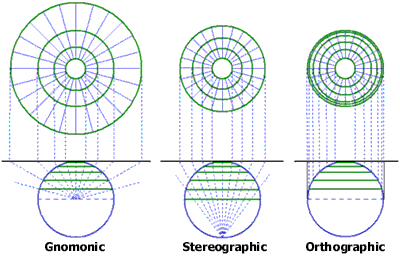
The Gnomonic projection views surface data from the center of the earth, the Stereographic projection views it from one pole to the opposite pole, and the Orthographic projection views the earth from an infinite point in space. The parallels for the projections at the top of the graphic are spaced 10 degrees apart.
Why are some northern hemisphere parallels missing in the Gnomonic map projection?
Because the map projection's plane and the equator are parallel, the projected line for the equator will never intersect the plane. The map projection is theoretically unlimited.
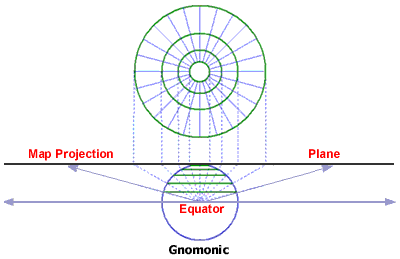
Cartographers limit the map projection when features become unacceptably distorted (for the northern hemisphere, this is about 30 degrees north).