c

The Lambert Conformal Conic projection was also developed by Johann Lambert around 1772. A conic projection that preserves shape (as its name implies), the projection wasn't appreciated for nearly a century after its invention.

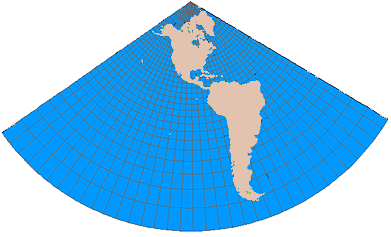
In a Lambert Conformal Conic map projection, latitude lines are unequally spaced arcs that are portions of concentric circles. Longitude lines are actually radii of the same circles that define the latitude lines. Poles are represented as single points. Notice the extreme distortion of the features farthest away from North America.

The Lambert Conformal Conic projection is one of the best projections for middle latitudes with an east–west orientation. It portrays shape more accurately than area and is common in many maps and geographic databases for North America. The State Plane Coordinate System, used throughout the United States, uses this projection for most state zones that are spread east to west.

The Lambert Conformal Conic projection can use a single latitude line as its point of contact (a tangent line), or the cone can intersect the earth's surface along two lines, called secants. Along these two lines there is no distortion, but distortion does occur as the distance from the secants increases.

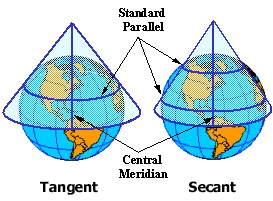
Distortion increases as you move away from the secant lines and the central meridian.

More about Johann Lambert

Johann Heinrich Lambert lived during a time when mathematics was expanding at a phenomenal rate. It was early in this period, known as the Mathematical Age of Enlightenment, that Isaac Newton lived and calculus was invented. Nearly a century later, Lambert used calculus so extensively that, without it, many of his advances in cartography probably could not have been made.

Johann Heinrich Lambert.

Lambert was born August 26, 1728, in Mulhausen, Alsace, to a tailor's family. Although his formal schooling ended at the age of twelve, he continued his quest for knowledge by teaching himself mathematics, astronomy, and physics. He soon developed a reputation as a child prodigy and mathematical genius. Before the age of twenty he was an expert in mathematics and philosophy, and fluent in five languages. In 1765, Frederick the Great appointed him Chief Counselor for Construction, where he worked on the Directing Committee of Frederick's Academy with Leonhard Euler and Joseph-Louis Lagrange, both known for their contributions to mathematics and map projections.

Many of his projections are still in use today and most carry his name, although he never actually named any of them. The most prominent of them are:

· Lambert Conformal Conic

· Lambert Cylindrical

· Lambert Conical Equal Area

· Lambert Azimuthal Equal Area

· Transverse Mercator

· Transverse Cylindrical Equal Area

Even this incomplete list shows how prolific he was. To say his influence is felt today through all the geosciences would be an understatement. The Lambert Conformal Conic, Transverse Mercator, and Lambert Azimuthal are three of the most common map projections used today and are applied daily in a variety of GIS applications.

Lambert died on September 25, 1777. An expert in many fields, he is probably best known today for his contributions to cartography. (Bugayevskiy 1998, Snyder 1987, and Tooley 1987).